Russell Towle (RT): The topmost image is a page from Steve Baer’s book?
HalfMoonBayMemories (HMBM) It’s from an old copy of the Whole Earth Catalog. An article about Buckminister Fuller, entitled Zome or Dome?
RT: I used to have that, maybe still have it somewhere. The polyhedron depicted on lower right is the Archimedean Truncated Icosidodecahedron, and is a zonohedron, a convex polyhedron bounded entirely by zonogons. Lower left is a “polar projection” of a polar zonohedron, the zonohedra I studied for so many years.
Now, so far as zonogons, and zonohedra, generalize to space of n dimensions and you have zonotopes. Always convex, always centrally symmetrical. Hypercubes, or n-cubes, including the square and the cube
for n=2 and n=3, are all zonotopes. The 4-cube or tesseract is bounded by eight 3-cubes; there is a relentless parallelism: each 3-cube has an equal and opposite 3-cube, each square face an equal and opposite square face, and the same with edges and vertices. This tesseract projects most symmetrically into three dimensions as Kepler’s Rhombic Dodecahedron. It so happens that this Dodecahedron can be dissected into four equal rhombic hexahedra in two ways; these are projections of the bounding 3-cubes of the tesseract.
It is all dimensional analogy which allows one to grasp these things. The master of such analogy was one Alicia Boole Stott of England, over a century ago. She was a younger daughter of the algebraist George
Boole of Boolean Algebra, which is the foundation of computer processors. But her father died when she was four, so her gift with the geometry of higher Euclidean spaces was not due to her father’s tutelage. Professor Coxeter provides an interesting little biography of her in his “Regular Polytopes.”
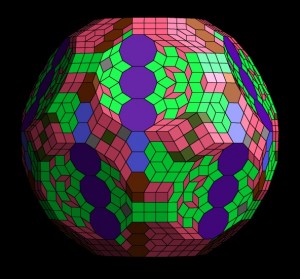
Well. Hypercubes fill their n-spaces, they close-pack. One might guess that their shadows, cast into 3-space, also close-pack. This is not usually true. For instance, the Archimedean Truncated Icosidodecahedron is an orthogonal shadow of a 15-cube, and does not close-pack to fill the 3-space. But one can contrive tilings in which mixtures of this zonohedron with other zonohedra *do* close-pack to
fill a 3-space. An example is attached. In this case the multitudes of zonohedra, colored according to their differing volumes, close-pack to build up a larger Truncated Icosidodecahedron. I try to illustrate such tilings on my YouTube channel.
R
Email Russell Towle ([email protected])